
For t 0, let f (t) be given and assume the function satisfies certain conditions to be stated later on.

Inverse Laplace Transform by Convolution Theorem: If then, 2. Rather than perform what can be a complicated integration, a table is provided with some of the most common transforms already completed: Transform Table. Inverse Laplace Transform: then is called inverse Laplace Transform of. The next step of transforming a linear differential equation into a transfer function is to reposition the variables to create an input to output representation of a differential equation. Laplace transform is the integral transform of the given derivative function with real variable t to convert into a complex function with variable s. The Laplace Transform is Linear If a is a constant and f and gare functions, then For example, by the above property (1) As an another example, by property (2) L(e5t+cos(3t)) L(e5t)+L(cos(3t)) 1 s5 + s s2+9 ,s>5. Laplace Transform of Derivative: then Laplace Transform of Bessel’s function:, where called Bessel’sfunction. Let us rewrite the transformation table to highlight the inverse Laplace transform operator instead. State the formula that you are using, as well as the value of any constants as your work for these problems: Please show neat work and steps for all three parts of the question. Inverse Laplace Transform Formula of Common Functions. (because, in applications, these are typically functions of time). Use a Laplace transform chart to compute the transforms of the following functions. F(s) is always the result of a Laplace transform and f(t) is always the result of an Inverse Laplace transform, and so, a general table is actually a table of. Note that the functions f(t) and F(s) are defined for time greater than or equal to zero. and t is (almost) always used as the variable in the formulas for these functions. Then, one transforms back into t-space using Laplace transform tables and the.
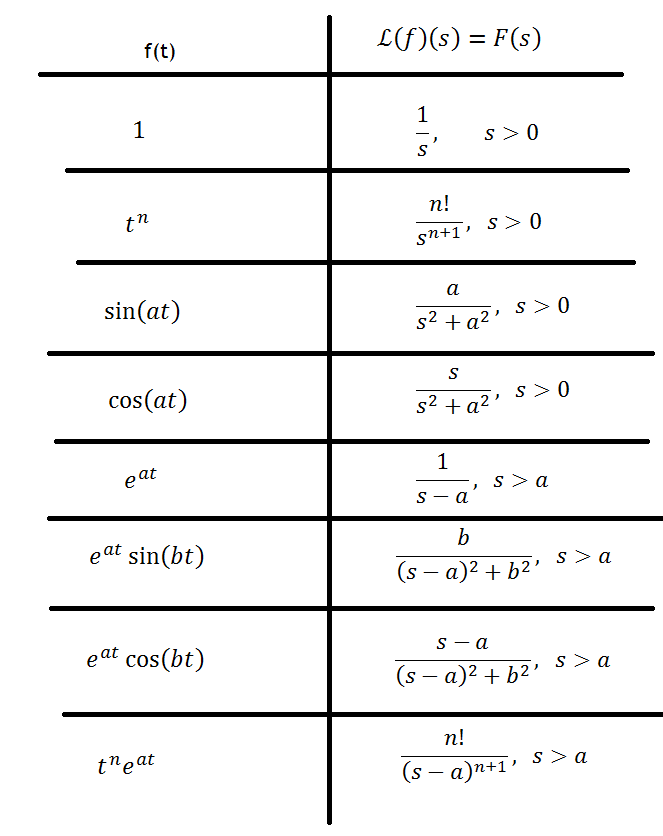
The Laplace transform is a mathematical tool which is used to convert the differential equation in time domain into the algebraic equations in the frequency domain or s-domain.Laplace Transform Table f(t) in Time Domain Typically, the algebraic equation is easy to solve for Y(s) as a function of s. An inverse Laplace transform table involving fractional and irrational operations is collected in Table A.2 (see 9, 35).


 0 kommentar(er)
0 kommentar(er)
